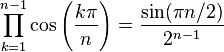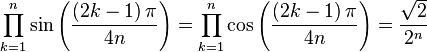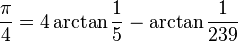Mix Max Nutty Bag: September 2015

Saturday, September 5, 2015

sherif monem

Humans could only survive on Mars for 68 days

Humans could only survive on Mars for 68 days according to a new study which throws doubt on ambitions to colonise the Red Planet.

Scientists at the Massachusetts Institute of Technology (MIT) concluded that with current technology a permanent settlement on Mars is "not feasible".

They analysed the Dutch-based Mars One project which is aiming to colonise the planet starting in 2024.

Mars One wants to send a group of people on a one-way trip and film the project for a reality television show.

^{Nasa's Mars Curiosity rover}

Tuesday, September 1, 2015

Algebra and Calculus Robert Blitzer

Overview

This book presents the traditional content of Precalculus in a manner that answers the age-old question of 'When will I ever use this?' Highlighting truly relevant applications, this book presents the material in an easy to teach from/easy to learn from approach. KEY TOPICS Chapter topics include equations, inequalities, and mathematical models; functions and graphs; polynomial and rational functions; exponential and logarithmic functions; trigonometric functions; analytic trigonometry; systems of equations and inequalities; conic sections and analytic geometry; and sequences, induction, and probability. For individuals studying Precalculus.

Identities without variables

The curious identity known as Morrie's law

\cos 20^\circ\cdot\cos 40^\circ\cdot\cos 80^\circ=\frac{1}{8}

is a special case of an identity that contains one variable:

\prod_{j=0}^{k-1}\cos(2^j x)=\frac{\sin(2^k x)}{2^k\sin(x)}.

Similarly:

\sin 20^\circ\cdot\sin 40^\circ\cdot\sin 80^\circ=\frac{\sqrt{3}}{8}.

The same cosine identity in radians is

\cos\frac{\pi}{9}\cos\frac{2\pi}{9}\cos\frac{4\pi}{9} = \frac{1}{8},

Similarly:

\tan 50^\circ\cdot\tan 60^\circ\cdot\tan 70^\circ=\tan 80^\circ.

\tan 40^\circ\cdot\tan 30^\circ\cdot\tan 20^\circ=\tan 10^\circ.

The following is perhaps not as readily generalized to an identity containing variables (but see explanation below):

\cos 24^\circ+\cos 48^\circ+\cos 96^\circ+\cos 168^\circ=\frac{1}{2}.

Degree measure ceases to be more felicitous than radian measure when we consider this identity with 21 in the denominators:

\begin{align} & \cos\left( \frac{2\pi}{21}\right) + \cos\left(2\cdot\frac{2\pi}{21}\right) + \cos\left(4\cdot\frac{2\pi}{21}\right) \\[10pt] & {} \qquad {} + \cos\left( 5\cdot\frac{2\pi}{21}\right) + \cos\left( 8\cdot\frac{2\pi}{21}\right) + \cos\left(10\cdot\frac{2\pi}{21}\right)=\frac{1}{2}. \end{align}

The factors 1, 2, 4, 5, 8, 10 may start to make the pattern clear: they are those integers less than 21/2 that are relatively prime to (or have no prime factors in common with) 21. The last several examples are corollaries of a basic fact about the irreducible cyclotomic polynomials: the cosines are the real parts of the zeroes of those polynomials; the sum of the zeroes is the Möbius function evaluated at (in the very last case above) 21; only half of the zeroes are present above. The two identities preceding this last one arise in the same fashion with 21 replaced by 10 and 15, respectively.
Other cosine identities include:^[38]

2\cos \frac{\pi}{3}=1,

2\cos \frac{\pi}{5} \times 2\cos \frac{2\pi}{5}=1,

2\cos \frac{\pi}{7} \times 2\cos \frac{2\pi}{7}\times 2\cos \frac{3\pi}{7}=1,

and so forth for all odd numbers, and hence

\cos \frac{\pi}{3}+\cos \frac{\pi}{5} \times \cos \frac{2\pi}{5}+\cos \frac{\pi}{7} \times \cos \frac{2\pi}{7} \times \cos \frac{3\pi}{7}+ \dots =1.

Many of those curious identities stem from more general facts like the following:^[39]

\prod_{k=1}^{n-1} \sin\left(\frac{k\pi}{n}\right) = \frac{n}{2^{n-1}}

and

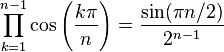
\prod_{k=1}^{n-1} \cos\left(\frac{k\pi}{n}\right) = \frac{\sin(\pi n/2)}{2^{n-1}}

Combining these gives us

\prod_{k=1}^{n-1} \tan\left(\frac{k\pi}{n}\right) = \frac{n}{\sin(\pi n/2)}

If n is an odd number (n = 2m + 1) we can make use of the symmetries to get

\prod_{k=1}^{m} \tan\left(\frac{k\pi}{2m+1}\right) = \sqrt{2m+1}

The transfer function of the Butterworth low pass filter can be expressed in terms of polynomial and poles. By setting the frequency as the cutoff frequency, the following identity can be proved:

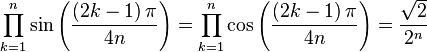
\prod_{k=1}^{n} \sin\left(\frac{\left(2k-1\right)\pi}{4n}\right) = \prod_{k=1}^{n} \cos\left(\frac{\left(2k-1\right)\pi}{4n}\right) = \frac{\sqrt{2}}{2^{n}}

Computing π[edit]

An efficient way to compute π is based on the following identity without variables, due to Machin:

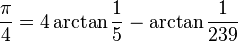
\frac{\pi}{4} = 4 \arctan\frac{1}{5} - \arctan\frac{1}{239}

or, alternatively, by using an identity of Leonhard Euler:

\frac{\pi}{4} = 5 \arctan\frac{1}{7} + 2 \arctan\frac{3}{79}

or by using Pythagorean Triples:

\pi = \arccos\frac{4}{5} + \arccos\frac{5}{13} + \arccos\frac{16}{65} = \arcsin\frac{3}{5} + \arcsin\frac{12}{13} + \arcsin\frac{63}{65}.

Mix Max Nutty Bag

Monday, September 28, 2015

Hunger and Poverty in Africa Picture Woman and Child